Question

What is the sum of the first 37 terms of the arithmetic sequence?

−27,−21,−15,−9,...



Enter your answer in the box.

Answer 1

The sum of the first 37 terms of the arithmetic sequence is 2997.

Explanation:

Arithmetic sequence concepts:

The general rule of an arithmetic sequence is the following:

`a_(n+1) = a_(n) + d`

In which d is the common diference between each term.

We can expand the general equation to find the nth term from the first, by the following equation:

`a_(n) = a_(1) + (n-1)*d`

The sum of the first n terms of an arithmetic sequence is given by:

`S_(n) = (n(a_(1) + a_(n)))/(2)`

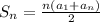
In this question:

`a_(1) = -27, d = -21 - (-27) = -15 - (-21) = ... = 6`

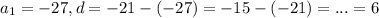
We want the sum of the first 37 terms, so we have to find
`a_(37)`

`a_(n) = a_(1) + (n-1)*d`

`a_(37) = a_(1) + (36)*d`

`a_(37) = -27 + 36*6`

`a_(37) = 189`

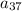
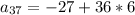
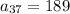
Then

`S_(37) = (37(-27 + 189))/(2) = 2997`

The sum of the first 37 terms of the arithmetic sequence is 2997.