Answer:
Correct answer is option D) Construct the bisector of an angle.
Explanation:
Angle bisector of an angle is a line that divides the angle in two equal parts.
A Line BD is called the angle bisector of
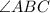 if
if
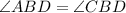
Please refer to image attached.
As per the question image, if the line PW is joined it will be the angle bisector of
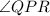 .
.
Steps of construction of angle bisector:
1. Draw the angle QPR.
2. Open compass and make an arc that will cut the two lines on points Q and R respectively as shown in question figure.
3. With the same compass opening, put the compass on Q and make an arc in the area between
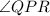 .
.
4. Draw an arc keeping the compass on R to cut the previous arc on point W.
Line joining the points P and W is the angle bisector of
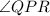 .
.
So, Correct answer is option D) Construct the bisector of an angle.