Answer:
Explanation:
We will use the area of the circle to find the radius of the circle since that's all that that is useful for! If the area of the circle is 56 cm squared, then
 and
and
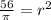 and
and
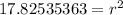 so
so
r = 4.222 cm
Now that we know that, we will use that. But first, if we have a square circumscribed in a circle, then we know that from the center of the circle to any of the vertices of the square is the radius of the circle, 4.222. If we split that square into 4 triangles, pull one of those triangles out, we have a right triangle...45-45-90 to be specific. Just for the sake of argument, put the 90 degree angle as the vertex angle of that right triangle and then split THAT triangle in half by dropping an altitude from the vertex angle to the base. We still have a 45-45-90 triangle, but now the vertex angle is 45, one of the base angles is 90, and the other base angle is 45. The hypotenuse of that triangle is the length of the radius we just found. If the base angle is 45 and the hypotenuse is 4.222, we can use right triangle trig to find the length of the base of that triangle which, consequently, is half of the length of x, our unknown.
 and
and
4.222 sin(45) = x so
x = 2.9854
But don't forget that that x is only half the length of one of the sides of the square. So multiply it by 2 to get that the side length, which is x, is
x = 5.97