Question

Find the coordinates of the other endpoint of the​ segment, given its midpoint and one endpoint.​ (Hint: Let​ (x,y) be the unknown endpoint. Apply the midpoint​ formula, and solve the two equations for x and​ y.)

Midpoint (-15,2) endpoint (-12,11)
What is the other endpoint?

Answer 1

The other endpoint of the​ segment is
`(-18,-7)`.

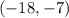Explanation:

The midpoint of the points
`(x_1,y_1)` and
`(x_2,y_2)` is given by the following formula:

`(x_m,y_m)=((x_1+x_2)/(2), (y_1+y_2)/(2) )`

where
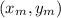`(x_m,y_m)` = coordinates of the midpoint.

We know that the midpoint is (-15, 2) and an endpoint is (-12, 11). Substituting the information we have gives:

`(-15,2)=((x_1-12)/(2), (y_1+11)/(2) )`

To find
`x_1` we need to solve this equation:

`-15=(x_1-12)/(2) \\\\(x_1-12)/(2)=-15\\\\(2\left(x_1-12\right))/(2)=2\left(-15\right)\\\\x_1-12=-30\\\\x_1-12+12=-30+12\\\\x_1=-18`

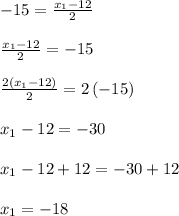
and to find
`y_1` we need to solve this equation:

`2= (y_1+11)/(2) \\\\(y_1+11)/(2)=2\\\\(2\left(y_1+11\right))/(2)=2\cdot \:2\\\\y_1+11=4\\\\y_1=-7`

The other endpoint of the​ segment is
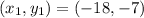`(x_1,y_1)=(-18,-7)`.