Question

The following function is continuous at all points in the domain of real numbers. What is the value of n?

f(x)= 2x+2, if x > n
4x, if x
`\leq` n
A. -1
B. 0
C. 1/2
D.1

Answer 1

1. A, -1

2. D, 1

3. B, It is discontinuous because there is a value a for which f(a) is not defined 4. D, It is discontinuous because there is a value a such that lim f(x) does not exist

Answer 2

D. 1

Explanation:

To solve this problem, we just need to find the common point of these linear function, beacuse they form a piecewise function which is continuous according to the problem, that means they are related by one common point.

Let's solve the following expression
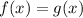`f(x)=g(x)`, where
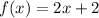`f(x) =2x+2` and
`g(x)=4x`. So,

`2x+2=4x\\2=4x-2x\\2x=2\\x=1`

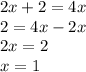
Which means
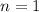`n=1`, that is, if the n is greater than 1, then the function is defined by
`f(x)`, if the n is least or equal than 1, then the functioni is defined by
`g(x)`

Therefore, the right answer is D.