Question

Prove that for any natural n the number 3^(n+4)−3^n is divisible by 16.

Answer 1

Proved (See Explanation)

Explanation:

Show that 3ⁿ⁺⁴ - 3ⁿ is divisible by 16.

This is done as follows

`(3^(n+4) - 3^n)/(16)`

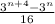
From laws of indices;

aᵐ⁺ⁿ = aᵐ * aⁿ.

So, 3ⁿ⁺⁴ can be written as 3ⁿ * 3⁴.

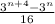`(3^(n+4) - 3^n)/(16)` becomes

`(3^n * 3^4 - 3^n)/(16)`

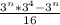
Factorize

`(3^n(3^4 - 1))/(16)`

`(3^n(81 - 1))/(16)`

`(3^n(80))/(16)`

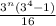
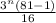
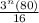
3ⁿ * 5

5(3ⁿ)

The expression can not be further simplified.

However, we can conclude that when 3ⁿ⁺⁴ - 3ⁿ is divisible by 16, because 5(3ⁿ) is a natural whole number as long as n is a natural whole number.