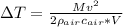Complete Question
The complete question is shown on the first uploaded image
Answer:
The expression for the change in the air temperature is
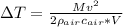
Step-by-step explanation:
From the question we are told that
The mass of the train is M
The speed of the train is v
The volume of the station is V
The density of air in the station is
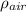
The specific heat of air is
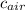
The workdone by the break can be mathematically represented as
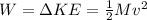
Now this is equivalent to the heat transferred to air in the station
Now the heat capacity of the air in the station is mathematically represented as
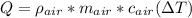
Now Since this is equivalent to the workdone by the breaks we have that
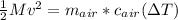
=>