Question

Ms. Nina wants to buy candy mix for $1.00 per pound. If she already bought 50 pounds of candy for $1.20 per pound, how many pounds that costs 80 cents per pound must she buy?

Answer 1

She must buy 50 pounds of the other candy in order to have a mix that costs $1 per pound.

Explanation:

Her goal is to have a candy mix that has a cost of $ 1.00 per pound, therefore the relation
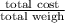`\frac{\text{total cost}}{\text{total weigh}}` must be equal to 1. She already bought a total of 50 pounds of a $1.2 per pound candy, therefore she spent:

`\text{spent first candy} = 50*1.2 = 60`

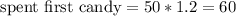
She wants to buy "x" of a 80 cents per pound candy, therefore if we sum it's weigh "x" with 50 pounds, then it must be equal to it's value summed with 60. We have:

`50 + x = 60 + 0.8*x\\x - 0.8*x = 60 - 50\\0.2*x = 10\\x = (10)/(0.2) = 50\\`

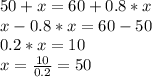
She must buy 50 pounds of the other candy in order to have a mix that costs $1 per pound.