Answer:
a) 60.4%; 18.42 kg/s
b) 37.8% ; 35.4 kg/s
Step-by-step explanation:
a) at an isentropic efficiency of 100%.
Let's first find the exit temperature of the compressor T2, using the formula:
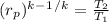
Solving for T2, we have:
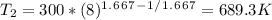
Let's now find the work dine by the compressor.
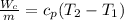
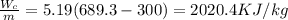
The actual work done by the compressor =
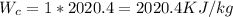
Let's find the temperature at the exit of the turbine, T4
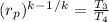
Solving for T4, we have:
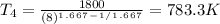
Let's find the work done by the turbine.


The actual work done by the turbine:
= 1 * 5276.6 = 5276.6 KJ/kg
Let's find the regeneration temperature, using the formula:
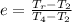
Substituting figures, we have:
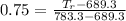
![T_r = [0.75(783.3 - 689.3)] + 689.3 = 759.8](https://img.qammunity.org/2021/formulas/engineering/college/sujo30b4ysv7mmsv2i9gwzy3hk9la2u352.png)
Let's calculate the heat supplied.
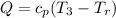
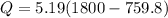
Q = 5388.2 kJ/kg
For thermal efficiency, we have:
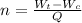
Substituting figures, we have:
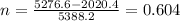
0.604 * 100 = 60.4%
For mass flow rate:
Let's use the formula:

Wnet = 60MW = 60*1000
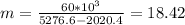
b) at an isentropic efficiency of 80%.
Let's now find the work done by the compressor.
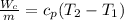
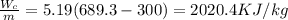
The actual work done by the compressor =
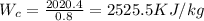
Let's find the work done by the turbine.

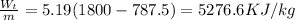
The actual work done by the turbine:
= 0.8 * 5276.6 = 4221.2 KJ/kg
Let's find the exit temperature of the compressor T2, using the formula:
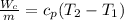
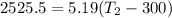
Solving for T2, we have:
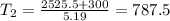
Let's find the temperature at the exit of the turbine, T4

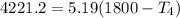
Solving for T4 we have:
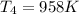
Let's find the regeneration temperature, using the formula:
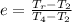
Substituting figures, we have:
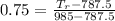
![T_r = [0.75(958 - 787.5)] + 787.5 = 935.5 K](https://img.qammunity.org/2021/formulas/engineering/college/ltd4h1bwbpvt0xffsfzvhsfzgwzyyq7rac.png)
Let's calculate the heat supplied.
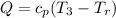
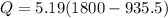
Q = 4486.2 kJ/kg
For thermal efficiency, we have:
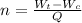
Substituting figures, we have:
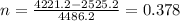
0.378 * 100 = 37.8%
For mass flow rate:
Let's use the formula:

Wnet = 60MW = 60*1000
