Answer:
9 and 11
Explanation:
The sum of the squares of two consecutive positive odd integers is 202.
Let first number be x and other number is (x+2).
According to question,
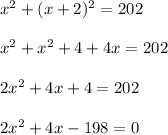
It is a quadratic equation. The solution of a quadratic equation is given by :

We have, a = 2, b = 4 and c = -198
So,
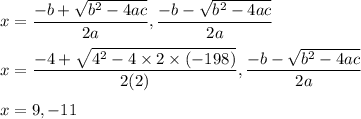
So, first positive odd integer is 9 and second one is (9+2)=11