Question

A metal ring 5.00 cm in diameter is placed between the north and south poles of large magnets with the plane of its area perpendicular to the magnetic field. These magnets produce an initial uniform field of 1.12 T between them but are gradually pulled apart, causing this field to remain uniform but decrease steadily at 0.220 T/s.

Required:
What is the magnitude of the electric field induced in the ring?

Answer 1

Ein: 2.75*10^-3 N/C

Step-by-step explanation:

The induced electric field can be calculated by using the following path integral:

`\int E_(in) dl=-(\Phi_B)/(dt)`

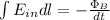
Where:

dl: diferencial of circumference of the ring

circumference of the ring = 2πr = 2π(5.00/2)=15.70cm = 0.157 m

ФB: magnetic flux = AB (A: area of the loop = πr^2 = 1.96*10^-3 m^2)

The electric field is always parallel to the dl vector. Then you have:

`E_(in)\int dl=E_(in)(2\pi r)=E_(in)(0.157m)`

Next, you take into account that the area of the ring is constant and that dB/dt = - 0.220T/s. Thus, you obtain:

`E_(in)(0.157m)=-A(dB)/(dt)=-(1.96*10^(-3)m^2)(-0.220T/s)=4.31*10^(-4)m^2T/s\\\\E_(in)=(4.31*10^(-4)m^2T/s)/(0.157m)=2.75*10^(-3)(N)/(C)`

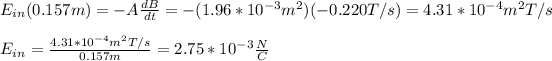
hence, the induced electric field is 2.75*10^-3 N/C