Question

Max has 5.50. He has eight more quarters than he does nickels. He had four times as many dimes as he has nickels. How many quarters, nickels, and dimes does max have?

Answer 1

There are 13 quarters, 5 nickels and 20 dimes.

Explanation:

Givens

• Max has $5.50.
• He has eight more quarters than he does nickels.
• He had four times as many dimes as he has nickels.

From these statements, we can deduce algebraical expressions to solve the problem. Let's call
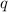`q` quarters,
`n` nickels and
`d` dimes.

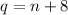`q=n+8` (8 more quarters than nickels)

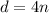`d=4n` (he has four times as many dimes as he has nickels).

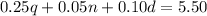`0.25q+0.05n+0.10d=5.50` (Max has a total of $5.50, one quartes is $0.25, one nickel is $0.05 and one dime is $0.10).

Let's replace the first two expressions into the third equation

`0.25(n+8)+0.05n+0.10(4n)=5.50\\0.25n+2+0.05n+0.40n=5.50\\0.70n=5.50-2\\n=(3.50)/(0.70)\\ n=5`

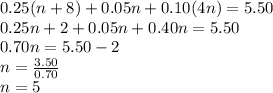
There are 5 nickels.

Now, we use this value to find the number of quarters

`q=5+8=13`

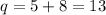
There are 13 quarters.

Also,
`d=4(5)=20`

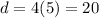
There are 20 dimes.

Therefore, there are 13 quarters, 5 nickels and 20 dimes.