Question

The table shows ordered pairs of the function . What is the value of y when ?

A 2-column table with 6 rows. The first column is labeled x with entries negative 3, negative 1, 1, 4, 8, 10. The second column is labeled y with entries 14, 10, 6, 0, question mark, negative 12.

–20
–8
8
48

Answer 1

-8

Explanation:

Answer 2

(B) -8

Explanation:

Given the 2-column table below:

`\left|\begin{array}cx&y\\--&--\\-3&14\\-1&10\\1&6\\4&0\\8&?\\10&-12\end{array}\right|`

Taking the pair (-3,14) and (-1,10)

`Slope, m=(10-14)/(-1-(-3)) =(-4)/(2) =-2`

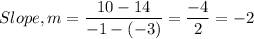
Taking the pair (1,6) and (4,0)

Slope,
`m=(0-6)/(4-1) =(-6)/(3) =-2`

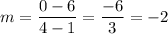
Since the slope is constant, the table represents a linear function whose slope is -2. Therefore:

Taking the pair (8,y) and (4,0)

Slope,
`m=(0-y)/(4-8) =-2`

`(-y)/(-4) =-2\\y=-2*4=-8`

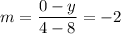
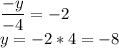
Therefore, the value of ? on the y-column is -8.