Answer:
take x=2.515834 and r=14.31386
Explanation:
Let us call the the lenght of the side of the square as x. The total lenght of the wire is 100. Note that if we have a square of side x, it has a perimeter of 4x. That means, that we need a wire of length 4x to build a square of side x. Note that if we take a wire of length 4x, we have that the remainder is 100-4x. We will use this to form a circle. As for the square, since we are using the wire of length 100-4x to build a circle, it must happen that the perimeter of the circle is 100-4x.
Recall that the perimeter of a circle of radius r is
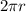 , so in this case , we have that
, so in this case , we have that
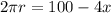 which implies that
which implies that
 .
.
Now, recall that the area of a square of side x is
 . Also, recall that the area of a circle of radius r is
. Also, recall that the area of a circle of radius r is
 . In our case, the area of the circle is given by
. In our case, the area of the circle is given by
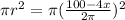
Also, we are given that the sum of the areas of both figures is 650. That is
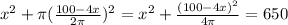
By substracting 650 on both sides and multiplying by
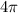 this equation is equivalent to
this equation is equivalent to
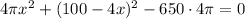
if we expand
 and then group each term of x, x^2 we get :
and then group each term of x, x^2 we get :

Recall that given a cuadractic equation of the form
 the solution is given by
the solution is given by
![x = \frac{-b \pm \sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2021/formulas/mathematics/high-school/blzih2n4birlry74b98s5wt2blugjf58n4.png)
In this case, the existence of a real solution is given by the expression
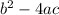 . For it to have a real solution, it must happen that
. For it to have a real solution, it must happen that
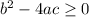
In this case, b=-800, c = 100^2-650\cdot 4\pi, a = 4\pi+16[/tex]
For this values, we get that
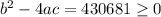 , so the is a solution for our problem.
, so the is a solution for our problem.
In this case,
![x = \frac{-b - \sqrt[]{b^2-4ac}}{2a}= 2.515834](https://img.qammunity.org/2021/formulas/mathematics/high-school/pl8vef0codl74qootq2qwmhsxrj6e1zl3d.png)
and
![x = \frac{-b + \sqrt[]{b^2-4ac}}{2a}= 25.4891](https://img.qammunity.org/2021/formulas/mathematics/high-school/4f01zqv6tknqj1kwpn86phd5u2jpfgk4h3.png) are the two possible solutions for this problem. We can check that this values of x fulfill our restrictions. Note that if x=25.4891, by replacing in the value of r, we get that r=-0.3113853. Since r is the radius of the circle, it must be positive. Hence, x=25.4891 is discarted.For x=2.515834 we get a value of r=14.31386, so this is the answer
are the two possible solutions for this problem. We can check that this values of x fulfill our restrictions. Note that if x=25.4891, by replacing in the value of r, we get that r=-0.3113853. Since r is the radius of the circle, it must be positive. Hence, x=25.4891 is discarted.For x=2.515834 we get a value of r=14.31386, so this is the answer