Answer:
Explanation:
The height of an object launched upward from ground level is given by the function s(t) = -4.9t² +7.8t
when the object reach the ground then s(t) = 0
So; -4.9t² +7.8t = 0
-4.9t² +7.8t + 0 = 0
By using the quadratic equation
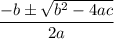
where; a = -4.9 ; b = 7.8 and c = 0
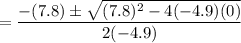
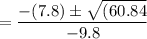
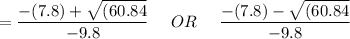
=0 OR 1.592
Hence; the distance of the object before it hits the ground after launch is t = 1.592 seconds