Question

Members of a soccer team suspect the coin used for the coin toss at the beginning of their games is unfair. They believe it turns up tails less often than it should if it were fair. The coach of the team decides to flip the coin 100 times and count the number of tails. His trial results in 35 tails. He decides to carry out a significance test. What is the p-value he obtains and the general conclusion that can be made at a 99% significance level?

A.) The p-value is 0.0013. He should reject the null in favor of the alternative.

B.) The p-value is 0.0013. He should fail to reject the null.

C.) The p-value is 0.9987. He should reject the null in favor of the alternative.

D.) The p-value is 0.9987. He should fail to reject the null.

E.) There is not enough information provided to calculate the p-value and make a conclusion.

Answer 1

The p-value is 0.0013. He should reject the null in favor of the alternative.

Explanation:

We are given that Members of a soccer team suspect the coin used for the coin toss at the beginning of their games is unfair. They believe it turns up tails less often than it should if it were fair.

The coach of the team decides to flip the coin 100 times and count the number of tails. His trial results in 35 tails.

Let p = proportion of occurrence of tail.

SO, Null Hypothesis,
`H_0` : p = 0.50 {means that it turns up tails equal to that it should if it were fair}

Alternate Hypothesis,
`H_A` : p < 0.50 {means that it turns up tails less often than it should if it were fair}

The test statistics that would be used here One-sample z-test for proportions;

T.S. =
`\frac{\hat p-p}{\sqrt{( p(1-p))/(n) } }` ~ N(0,1)

where,
`\hat p` = sample proportion of tails occurrence =
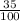`(35)/(100)` = 0.35

n = sample of trails = 100

So, the test statistics =
`\frac{0.35-0.50}{\sqrt{(0.50(1-0.50))/(100) } }`

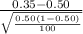
= -3

The value of z test statistics is -3.

Also, P-value of the test statistics is given by;

P-value = P(Z < -3) = 1 - P(Z
`\leq` 3)

= 1 - 0.9987 = 0.0013

Since, the P-value of test statistic is less than the level of significance as 0.0013 < 0.01, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that it turns up tails less often than it should if it were fair.