Answer:
The final pressure inside the container is 107.7 atm
Step-by-step explanation:
Given;
volume of the closed container = 7.20 L
mass of liquid helium in the container = 10.9 g
initial temperature of the container = 3.00 K
initial pressure of the container = 1.00 atm.
final temperature of the container = 293 K
Number of moles of He in the container = 10.9 / 4 = 2.725 moles
At 273˚K and 1 atm pressure (STP), the volume of 1 mole of a gas = 22.4 L
Volume of 2.725 moles of He at STP = 2.725 x 22.4 = 61.04 L
Volume of 2.725 moles of He at 3 K and 1 atm = (3/273) x 61.04 = 0.671 L
Thus, volume of He = 0.671 L
Volume of air in the container = 7.2 L – 0.671 L = 6.529 L
Since, the liquid helium evaporated, we only have air left in the container,
thus, final volume of the container, V₂ = 6.529 L
Initial volume of the container, V₁ = 7.20 L
Initial pressure of the in the container, P₁ = 1 atm
initial temperature of the container, T₁ = 3 K
Final temperature of the container, T₂ = 293 K
Final pressure of the container, P₂ = ?
Apply general gas law, in order to estimate P₂
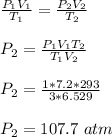
Therefore, the final pressure inside the container is 107.7 atm