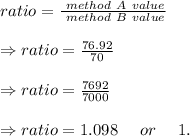Question

Method A assumes simple interest over final fractional periods, while Method B assumes simple discount over final fractional periods. The annual effective rate of interest is 20%. Find the ratio of the present value of a payment to be made in 1.5 years computed under method A to that computed under Method B.

Answer 1

The answer is "1.1"

Step-by-step explanation:

In the case of a single Interest, the principal value is determined as follows:

`\ I = Prt \\\ A = P + I\\A = P(1+rt) \\\\A = amount \\P= principle\\r = rate\\t= time`

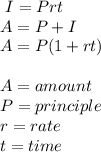
In case of discount:

`D = Mrt \\P = M - D \\P = M(1-rt)\\\\Where, D= discount \\M =\ Maturity \ value \\`

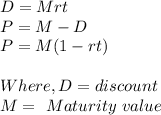
Let income amount = 100, time = 1.5 years, and rate =20 %.

Formula:

A = P(1+rt)

A =P+I

by putting vale in the above formula we get the value that is = 76.92, thus method A will give 76.92 value.

If we calculate discount then the formula is:

P = M(1-rt)

M = 100 rate and time is same as above.

`P = 100(1-0.2 * 1.5) \\P = 100 * (70)/(100) \\P = 70`

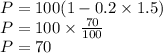
Thus Method B will give the value that is 70

calculating ratio value:

`ratio = \frac{\ method\ A \ value} {\ method \ B \ value}\\\\\Rightarrow ratio = (76.92)/(70)\\\\\Rightarrow ratio = (7692)/(7000)\\\\\Rightarrow ratio = 1.098 \ \ \ \ or \ \ \ \ 1.`