Answer: 0.00494.
Explanation:
Given : The proportion of the company's orders come from first-time customers.
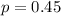
Sample size : n= 166
Now , the probability that the sample proportion is between 0.24 and 0.35 would be :-
![P(0.24<p<0.35)=P\left({\frac{0.24-0.45}{\sqrt{(0.45(1-0.45))/(166)}}<\frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}<\frac{0.35-0.45}{\sqrt{(0.45(1-0.45))/(166)}}}\right)\\\ \ \left[\because z=\frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}\right]](https://img.qammunity.org/2021/formulas/mathematics/college/uz7s0owgw472mpd3nsqxzrk6jbkyo62zus.png)
![=P(-5.44<z<-2.58)=P(z<-2.58)-P(z<-5.44)\\\\=1-P(z<2.58)-(1-P(z<5.44))\\\\=P(z<5.44)-P(z<2.58)\\\\=1-0.99506\ [\text{By z-value table}]\\\\=0.00494](https://img.qammunity.org/2021/formulas/mathematics/college/x8omzvdnv7ei3ku22vwg6fdiry9qtxdais.png)
Hence, the probability that the sample proportion is between 0.24 and 0.35 is 0.00494.