Question

A disk with a rotational inertia of 2.5 kg-m2 and a radius 1.1 m rotates on a frictionless fixed axis perpendicular to the disk faces and through its center. A force of 7.7 N is applied tangentially to the rim. The angular acceleration of the disk is _____ rad/s2. Round your answer to the nearest tenth.

Answer 1

3.4 rad/sec^2

Step-by-step explanation:

rotational inertia = 2.5 kg-m^2 radius = 1.1 m force = 7.7 N

t = rotational inertia * angular acceleration equation 1

also t = force * radius

therefore to calculate angular acceleration equation 1 becomes

f * r = inertia * angular acceleration hence

angular acceleration = f * r / inertia =
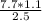`(7.7 * 1.1 )/(2.5)` 8.47 / 2.5 = 3.388 ≈ 3.4 rad/sec^2