Answer:
48.67% probability that the tires will fail within two years of the date of purchase
Explanation:
Exponential distribution:
The exponential probability distribution, with mean m, is described by the following equation:
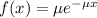
In which
 is the decay parameter.
is the decay parameter.
The probability that x is lower or equal to a is given by:
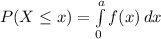
Which has the following solution:
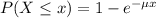
In this question:
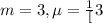
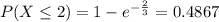
48.67% probability that the tires will fail within two years of the date of purchase