We have been given that the typical amount of sleep per night that adults get has a bell-shaped distribution with a mean of 7.5 hours and a standard deviation of 1.3 hours. Suppose last night you slept for 5 hours.
We will use z-score formula to solve our given problem as z-score tells a data point is how many standard deviation from the mean.
 , where,
, where,
z = z-score,
x = Random sample score,
 = Mean,
= Mean,
 = Standard deviation.
= Standard deviation.
Upon substituting our given values in z-score formula, we will get:

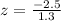
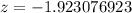
Upon rounding to two decimal places, we will get:
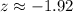
Therefore, you are
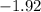 standard deviations from the mean or 1.92 standard deviation below mean.
standard deviations from the mean or 1.92 standard deviation below mean.