Question

Suppose a square has side length s. How could you use the formula for the area of a trapezoid to find the area of the square?

Answer 1

The area of a square can be found from the area of a trapezoid by replacing the parallel sides of the trapezoid and the height by the length of the side of the square

Explanation:

The area of a trapezoid is given as follows;

`A = (a + b)/(2) h`

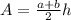
Where:

a and b = the parallel sides of the trapezoid

h = Height of the trapezoid

Therefore, whereby the trapezoid is a square of side s, we have;

a = b = s

h = s

Plugging in the values into the formula for finding the area of a trapezoid, we have;

`A = (s + s)/(2) * s = (2 \cdot s)/(2) * s= s * s = s^2 = Area \ of \, a \, square`

Therefore, the area of a square can be found from the area of a trapezoid by replacing the parallel sides of the trapezoid and the height by the length of the side of the square.