Answer:
3 feet.
Explanation:
- Base of the triangular pennant = 1.5 feet long.
- Area of the triangular pennant = 2.25 Square feet.
Now, we know that:
Area of a Triangle
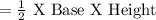
Substituting the given values, we have:

Therefore, the height of the pennant is 3 feet.