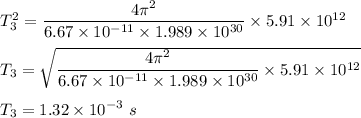Step-by-step explanation:
The orbital radius of the Earth is
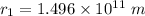
The orbital radius of the Mercury is
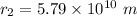
The orbital radius of the Pluto is
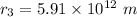
We need to find the time required for light to travel from the Sun to each of the three planets.
(a) For Sun -Earth,
Kepler's third law :
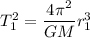
M is mass of sun,
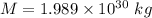
So,
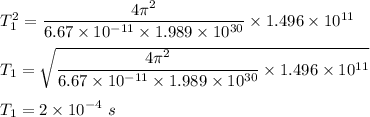
(b) For Sun -Mercury,

(c) For Sun-Pluto,