Question

Their vehicles was recorded for each sample. The sample proportions were used to construct the 95 percent confidence interval for a difference in population proportions (FWD minus AWD ) for satisfied owners. The interval is given as (−0.01,0.12) . A car company believes that the proportion of satisfied owners of AWD AWD vehicles differs from the proportion of satisfied owners of FWD FWD vehicles. Does the confidence interval provide evidence that this belief is plausible?

Answer 1

`-0.01 \leq p \leq 0.12`

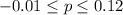
The confidence is 0.95 or 95% and the significance would be given by:
`\alpha=1-0.95=0.05`/

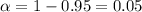For this case our confidence interval contains the value 0. So then we can conclude that we don't have significant differences in the the proportion of satisfied owners of AWD vehicles from the proportion of satisfied owners of FWD at the significance level used of 5%. So then the claim that the two proportions are different is incorrect with the results obtained

Explanation:

The confidence interval for the true proportion would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

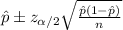
And for this case we have the following result:

`-0.01 \leq p \leq 0.12`

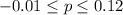
The confidence is 0.95 or 95% and the significance would be given by:
`\alpha=1-0.95=0.05`/

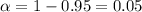For this case our confidence interval contains the value 0. So then we can conclude that we don't have significant differences in the the proportion of satisfied owners of AWD vehicles from the proportion of satisfied owners of FWD at the significance level used of 5%. So then the claim that the two proportions are different is incorrect with the results obtained