Question

Triangle A is a scaled version of triangle B. The dimensions of triangle B are three times the dimensions of triangle A. The area of triangle A is 24.5 sq cm. What is the area of triangle B?

Answer 1

The area of triangle B is
`2.72cm ^(2)`

Explanation:

We will use the principle of similar triangles to solve the problem.

Since triangle A is just a scale version of triangle B, we can say that the two triangles are similar.

The area scale factor is the square of the length scale factor.

Given the length scale factor as 3,

The area scale factor will be 3 X 3 = 9 sq. cm

(Area of triangle A / Area of triangle B) = (length of side A / Length of side B) square

`(24.5)/( area of B)= 3^(2)`

`(24.5)/(Area of B)= 9\\Area of B = (24.5)/(9)= 2.72 cm ^(2)`

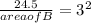
The area of triangle B is
`2.72cm ^(2)`