Answer:
45cm
Step-by-step explanation:
A converging mirror is also known as a concave mirror. For a concave mirror, its focal length and the image distance are both positive.
Using the mirror formula as shown to get the object distance;
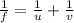
f is the focal length of the mirror
u is the object distance
v is the image distance
Given f = 30cm and v = 2u (The image formed is twice the size of the pencil)
Substituting this values in the formula to get u we have;
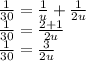
Cross multiplying we have;
2u = 90
Dividing both sides by 2;
2u/2 = 90/2
u = 45cm
The distance of the object from the mirror is 45cm