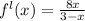Question

Drag each tile to the correct box. Not all tiles will be used.

Arrange the equations in the correct sequence to find the inverse of f(x) = y = 3x / 8 + x​

Answer 1

Inverse of f(x)

`f^(l) (x) = (8 x)/(3-x)`

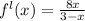
Explanation:

Explanation:-

Step(i):-

Given the function

`f(x) = (3 x)/(8+x)`

Given function is one-one and onto function

Hence f(x) is bijection function

`y = f(x) = (3 x)/(8+x)`

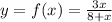
now cross multiplication, we get

( 8+x)y = 3 x

8 y + x y = 3 x

8 y = 3 x - x y

taking Common 'x' we get

x (3 - y) = 8 y

`x = (8 y)/(3-y)`

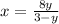
Step(ii):-

The inverse function

`x = (8 y)/(3-y) = f^(l)(y)`

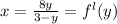
The inverse function of x

`f^(l)(x) = (8 x)/(3-x)`

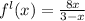
Final answer:-

Inverse of f(x)

`f^(l) (x) = (8 x)/(3-x)`