Answer:
It would take 74.5 minutes for the element to decay 17 grams.
Explanation:
The amount of element X after t minutes is given by the follwoing equation:

In which X(0) is the initial amount of the substance and r is the decay rate.
Half life of 14 minutes.
This means that

So

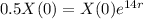


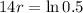

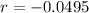
So

There are 680 grams of element X
This means that
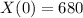

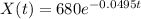
How long would it take the element to decay 17 grams
This is t for which X(t) = 17. So
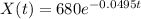
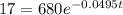
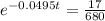
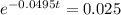
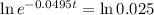
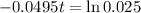

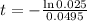
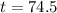
It would take 74.5 minutes for the element to decay 17 grams.