Final answer:
The 14th term of the geometric sequence is -1220703125.
Step-by-step explanation:
The given sequence is geometric because each term is obtained by multiplying the previous term by a constant ratio.
In this case, the ratio between each term is -5/1 = -5.
To find the 14th term, we can use the formula for the nth term of a geometric sequence:
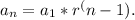
Substituting in the values from the sequence, we have -
a_14
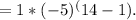
Simplifying, we get
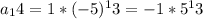
= -1 * 1220703125
= -1220703125.
Therefore, the 14th term of the geometric sequence is -1220703125.