Question

How many ways can you divide nine children into a team of 4, a team of 3, and a team

of 2?

Ana writes down all the numbers 1, 2, . . . , n, for some positive integer n. In doing so,
she writes a total of 600 digits. Find the positive integer n.

Answer 1

If we want to divide a group of N objects into K objects; we have

`c = (N!)/((N - K)!*K!)`

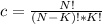
combinations

For the first group, N = 9 and K = 4

`c1 = (9!)/(5!*4!) = (9*8*7*6)/(4*3*2) = 126`

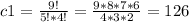
For the second group we have N = 5 (cause we already took 4 of them) and K = 3

`c2 = (5!)/(2!*3!) = 10`

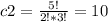
For the last group we have N =2 and K = 2, so there is only one possible combination, c3 = 1 then we have that the total number of combinations is:

C = c1*c2*c3 = 126*10*1 = 1260 possible divisions.

b)

numbers with one digit = 9, in those 9 numbers we have 9 digits.

numbers with two digits; (99 - 10 = 89) numbers, in those 89 numbers we have 2*89 = 178 digits, plus the 9 of before we have 178 + 9 = 183.

Now, for each number of 3 digits we have 3 digis obviusly, so we can write:

N*3 = 600 - 183

and N is the number of 3 digits number needed.

N = 317/3 = 139

So the 139-th number of 3 digits, this is:

100 + 139 - 1

The minus one goes because 100 is already a 3 digit number:

100 + 139 - 1 = 238

238 is the positive integer n.