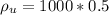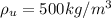Question

An object weigh 40N in air ,weigh 20N when submerged in water,and 30N when submerged in a liquid of unknown liquid density.what is the density of unknown of liquid?

Answer 1

The density is
`\rho_u =500 kg /m^3`

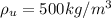
Step-by-step explanation:

From the question we are told that

The weight in air is
`W_a = 40 \ N`

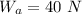
The weight in water is
`W_w = 20 \ N`

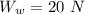
The weight in a unknown liquid is
`W_u = 30 \ N`

Now according to Archimedes principle the weight of the object in water is mathematically represented as

`W_w = W_a -m _w g`

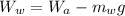
Where
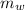`m_w` is he mass of the water displaced

substituting value

`m_w g = 40 -20`

`m_w g = 20 \ N --- (1)`

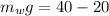
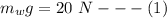
Now according to Archimedes principle the weight of the object in unknown is mathematically represented as

`W_u = W_a -m _u g`

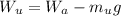
Where
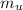`m_u` is he mass of the unknown liquid displaced

substituting value

`m_u g = 40 -30`

`m_u g = 10 \ N ---(2)`

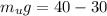
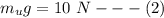
dividing equation 2 by equation 1

`(m_ug)/(m_wg) = (10)/(20)`

`(m_u)/(m_w) = (1)/(2)`

=>
`m_u = 0.5 m_w`

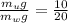
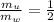
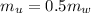
Now since the volume of water and liquid displaced are the same then

`\rho _u = 0.5 \rho_w`

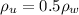
This because

`density = (mass)/(volume)`

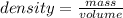
So if volume is constant

mass = constant * density

Where
`\rho_u` is the density of the liquid

and
`\rho_ w` is the density of water which is a constant with a value
`\rho_w = 1000 kg/m^3`

So

`\rho_u = 1000*0.5`

`\rho_u =500 kg /m^3`