Question

The probability distribution of the number of loaves of bread sold in a bakery in a week based on past data is given below. Identify the expected number of loaves of bread sold for one week.

Number of Loaves, n: 100 148 135 200
Prob. of n Loaves: 0.25 0.36 0.21 0.18

Answer 1

`E(X) = 100*0.25+ 148*0.36 + 135*0.21 + 200*0.18 = 142.63`

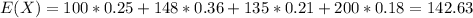
And for this case the expected value for this random variable is given by 142.63 and rounded to the nearest integer we got 143. And that represent the number of loaves of bread sold for one week

Explanation:

For this case we have the following distirbution given:

X: 100 148 135 200

P(X): 0.25 0.36 0.21 0.18

The expected value is given by:

`E(X) = \sum_(i=1)^n X_i P(X_i)`

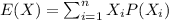
And replacing we got:

`E(X) = 100*0.25+ 148*0.36 + 135*0.21 + 200*0.18 = 142.63`

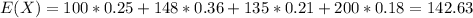
And for this case the expected value for this random variable is given by 142.63 and rounded to the nearest integer we got 143. And that represent the number of loaves of bread sold for one week