Answer:
Assume that this wavelength is measured in vacuum. The energy on each photon of this wave would be approximately
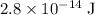 .
.
Step-by-step explanation:
The Planck-Einstein Relation relates the energy
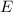 of a photon to its frequency
of a photon to its frequency
 :
:
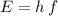 ,
,
where
 is Planck's Constant.
is Planck's Constant.
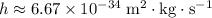 .
.
This question did not provide the frequency
 of this wave directly; the value of
of this wave directly; the value of
 needs to be calculated from the wavelength
needs to be calculated from the wavelength
 of this wave. Assume that this wave is travelling at the speed of light in vacuum:
of this wave. Assume that this wave is travelling at the speed of light in vacuum:
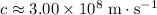 .
.
The frequency of this electromagnetic wave would be:
 .
.
Apply the Planck-Einstein Relation to find the energy of a photon of this electromagnetic wave:
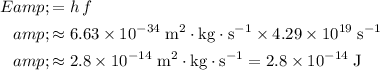 .
.
Note that combining the two equations above (
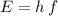 and
and
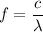 ) will give:
) will give:
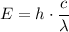 .
.
This equation is supposed to give the same result (energy of a photon of this wave given its wavelength and speed) in one step:
 .
.