Answer:
An adult ticket costs $10 and a child ticket costs $4.5.
Explanation:
This question can be solved using a system of equations.
I am going to say that:
x is the cost of an adult ticket.
y is the cost of a children ticket.
They sold 3 adult tickets and 8 children's tickets and made $66.
This means that
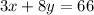
The next day they sold 8 adult tickets and 15 children's tickets and took in $147.50.
This means that
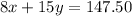
Solving the system:
I will use the addition method, multiplying the first equation by -8, the second by 3, and adding them. So
-24x - 64y = -528
24x + 45y = 442.5
-24x + 24x - 64y + 45y = -528 + 442.5
19y = 85.5
y = 85.5/19
y = 4.5
And
3x + 8y = 66
3x = 66 - 8y
3x = 66 - 8*4.5
3x = 30
x = 30/3
x = 10
An adult ticket costs $10 and a child ticket costs $4.5.