Answer:
11.34 units²
Explanation:
Area of a sector is expressed as
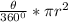
Before we can get the area of the circle, we need to find its radius. The radius of the circle can be derived from the equation of the circle given.
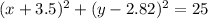
The general form of equation of a circle is given as
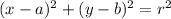 where r is the radius of the circle. Comparing the general equation to the given equation to get the radius r;
where r is the radius of the circle. Comparing the general equation to the given equation to get the radius r;
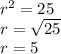
The radius of the circle is 5
Given the angle subtended by the sector of the circle to be 52°,
Area of the sector =
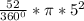
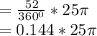

This gives the required area of the sector to nearest hundredth