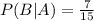Question

A bag contains 4 red, 7 blue and 5 yellow marbles. Event A is defined as drawing a yellow marble on the first draw and event B is defined as drawing a blue marble on the second draw. If two marbles are drawn from the bag, one after the other and not replaced, what is P(B|A) expressed in simplest form?

Answer 1

B

Explanation:

Answer 2

P(B|A) is the probability of drawing a blue marble on the second draw, given that a yellow marble was chosen on the first draw.

`P(B|A) = (7)/(15)`

Explanation:

P(B|A) is the conditional probability of event B happening when event A has happened.

In this question:

Event A: Drawing a yellow marble on the first draw.

Event B: Drawing a blue marble on the second draw.

P(B|A) is the probability of drawing a blue marble on the second draw, given that a yellow marble was chosen on the first draw.

Finding P(B|A):

Suppose a yellow marble was chosen on the first draw.

There are will be 4+7+4 = 15 marbles, of which 7 are blue. So

`P(B|A) = (7)/(15)`