Question

A culture of bacteria has an initial population of 450 bacteria and doubles every 4 hours. Using the formula P t = P 0 ⋅ 2 t d P t ​ =P 0 ​ ⋅2 d t ​ , where P t P t ​ is the population after t hours, P 0 P 0 ​ is the initial population, t is the time in hours and d is the doubling time, what is the population of bacteria in the culture after 3 hours, to the nearest whole number?

Answer 1

757

Explanation:

We use the model:
`P(t) = P_0\cdot 2^(t/d)`

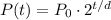
From the given information

• Initial Population of the bacteria culture,
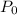  `P_0` =450
• Doubling Time, d=4 hours

On substitution of these into the model, we have:

`P(t) = 450\cdot 2^(t/4)`

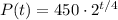
We want to determine the population of the bacteria culture, P(t) after 3 hours.

When t=3

`P(3) = 450\cdot 2^(3/4)\\=756.8\\\approx 757$ bacteria (to the nearest whole number)`

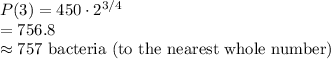
Therefore, the bacteria culture contains approximately 757 bacteria after 3 hours.