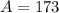Answer:
The area of the triangle is 173 squared meters.
Explanation:
An equilateral triangle has all sides equal to each other.
Finding the length of each side of the triangle.
Like I said before, in an equilateral triangle all the sides are equal. The perimeter of a triangle is every side added together. Since each side is equal, you will divide 60 (the perimeter) by 3 (the number of sides).

Each side is equal to 20 meters.
Finding the area of the triangle
To find the area of a triangle you need the formula:
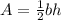
A is the area, b is the base, and h is the height.
b = 20
h = 17.3
 Multiply what is in the parenthesis first
Multiply what is in the parenthesis first
 Multiply 346 by 1
Multiply 346 by 1
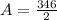 Divide 346 by 2
Divide 346 by 2