Answer:
The inequality which represents the possible values of w is w < 8.
Explanation:
A rectangle has two dimensions, the length(l) and the width(w).
The area of a rectangle is:

In this question, we have that:
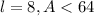
So
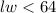
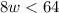
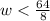
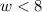
The inequality which represents the possible values of w is w < 8.