Answer:
The significance level is
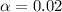 and
and
 we want to find the decision rule. Since is a bilateral test the critical values are:
we want to find the decision rule. Since is a bilateral test the critical values are:

And for this case the decision rule would be reject the null hypothesis if the calculated value is:
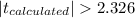
And for this case the calculated value is higher than 2.326 so then we have enough evidence to reject the null hypothesis at the significance level given.
Explanation:
Information given
n=900 represent the random sample mean
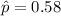 estimated proportion of the chips fail in the first 1000 hours of their use
estimated proportion of the chips fail in the first 1000 hours of their use
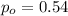 is the value to verify
is the value to verify
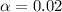 represent the significance level
represent the significance level
z would represent the statistic
System of hypothesis
We want to test if the actual percentage that fail is different from the stated percentage, the system of hypothesis are.:
Null hypothesis:
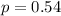
Alternative hypothesis:
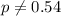
The statistic is given by:
 (1)
(1)
Replacing the info given we got:
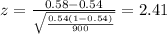
The significance level is
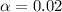 and
and
 we want to find the decision rule. Since is a bilateral test the critical values are:
we want to find the decision rule. Since is a bilateral test the critical values are:

And for this case the decision rule would be reject the null hypothesis if the calculated value is:
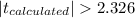
And for this case the calculated value is higher than 2.326 so then we have enough evidence to reject the null hypothesis at the significance level given.