Answer:
Minimum Sample size 'n' = 585
Explanation:
Explanation:-
Given Estimate of the population proportion as 58% ( = 0.58)
P = 0.58
Given margin of error, E, to be 4%
M.E = 4 % = 0.04
margin of error of the population proportion is determined by
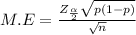
Z- score = 1.96

Cross multiplication , we get
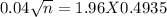
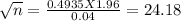
Squaring on both sides, we get
n = 584.7≅585
Conclusion:-
minimum Sample size 'n' = 585