Question

Birth weights in the United States are normally distributed with a mean of 3420 g and a standard deviation of 495 g. The Newport General Hospital requires special treatment for babies that are less than 2450 g (unusually light) or more than 4390 g (unusually heavy). What is the percentage of babies who do not require special treatment because they have birth weights between 2450 g and 4390 g

Answer 1

`P(2450<X<4390)=P((2450-\mu)/(\sigma)<(X-\mu)/(\sigma)<(4390-\mu)/(\sigma))=P((2450-3420)/(495)<Z<(4390-3420)/(495))=P(-1.96<z<1.96)`

And we can find this probability with this difference:

`P(-1.96<z<1.96)=P(z<1.96)-P(z<-1.96)`

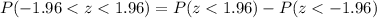
If we use the normal standard table or excel we got:

`P(-1.96<z<1.96)=P(z<1.96)-P(z<-1.96)=0.975-0.025=0.95`

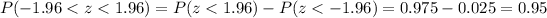
And that represent 95% of the data. so then the percentage below 2450 is 2.5% and above 4390 is 2.5 %

Explanation:

Let X the random variable that represent the birth weights of a population, and for this case we know the distribution for X is given by:

`X \sim N(3420,495)`

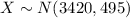
Where
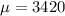`\mu=3420` and
`\sigma=495`

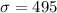
We are interested on this probability

`P(2450<X<4390)`

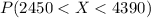
And we can use the z score formula given byÑ

`z=(x-\mu)/(\sigma)`

If we apply this formula to our probability we got this:

`P(2450<X<4390)=P((2450-\mu)/(\sigma)<(X-\mu)/(\sigma)<(4390-\mu)/(\sigma))=P((2450-3420)/(495)<Z<(4390-3420)/(495))=P(-1.96<z<1.96)`

And we can find this probability with this difference:

`P(-1.96<z<1.96)=P(z<1.96)-P(z<-1.96)`

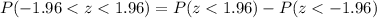
If we use the normal standard table or excel we got:

`P(-1.96<z<1.96)=P(z<1.96)-P(z<-1.96)=0.975-0.025=0.95`

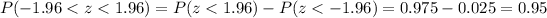
And that represent 95% of the data. so then the percentage below 2450 is 2.5% and above 4390 is 2.5 %