Answer:
The standard deviation of the age distribution is 6.2899 years.
Explanation:
The formula to compute the standard deviation is:
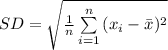
The data provided is:
X = {19, 19, 21, 25, 25, 28, 29, 30, 31, 32, 40}
Compute the mean of the data as follows:
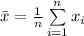
![=(1)/(11)* [19+19+21+...+40]\\\\=(299)/(11)\\\\=27.182](https://img.qammunity.org/2021/formulas/mathematics/college/y9vnpdc6h5c0ks9xi3eo0umqffamhahnei.png)
Compute the standard deviation as follows:
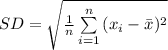
![=\sqrt{(1)/(11-1)* [(19-27.182)^(2)+(19-27.182)^(2)+...+(40-27.182)^(2)]}}\\\\=\sqrt{(395.6364)/(10)}\\\\=6.28996\\\\\approx 6.2899](https://img.qammunity.org/2021/formulas/mathematics/college/2r7csbzswyrecncxbh5op7mqj8e9g1mv6w.png)
Thus, the standard deviation of the age distribution is 6.2899 years.