Question

Please help !! how many solutions does this system have ?

Answer 1

It has one unique solution. Therefore, the correct answer is:B. One

The system of equations has one solution because the two lines represented by the equations are not parallel and will intersect at a single point.

Let's analyze the system of equations:

1.
`\(x + 4y = 9\)`

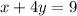
2.
`\(-2x + y = 0\)`

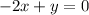
We can use various methods to determine the solutions, such as substitution or elimination. In this case, let's use the substitution method:

From equation (2), we can solve for y:

`\[y = 2x\]`

Now substitute this expression for y into equation (1):

`\[x + 4(2x) = 9\]`

Simplify:

`\[x + 8x = 9\]\\9x = 9\]x = 1\]`

Now substitute x = 1 back into the expression we found for y:

`\[y = 2(1) = 2\]`

So, the solution to the system is x = 1 and y = 2.

The system has one unique solution. Therefore, the correct answer is:

B. One

Answer 2

none it has no solutions