Question

Maya bought a new roll of tape.

The smallest circle has a radius of 0.75in. The tape is 0.25in away from the small circle. What is the area of just the tape part?

Answer 1

area of the tape part is 1.38 square inches.

Explanation:

From the question, the new roll of tape has the smallest circle of radius 0.75 inches and tape part which is 0.25 inches away from the small circle.

Since,

Area of a circle =
`\pi`
`r^(2)` ...................... (1)

Area of tape part = area of the roll - area of the small circle ................. (2)

Area of the small circle =
`\pi`
`r^(2)`

Given that r = 0.75 inches, then;

Area of tape part =
`\pi` (
`0.75^(2)`)

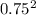= 0.5625
`\pi` square inches

Area of the roll =
`\pi`
`r^(2)`

radius, r, of the roll = radius of the small circle + distance of the tape away from the circle

= 0.75 + 0.25

= 1.0 inches

So that;

Area of the roll =
`\pi` ×
`(1)^(2)`

=
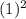
`\pi` square inches

Therefore from equation (2),

Area of tape part =
`\pi` - 0.5625
`\pi`

= 0.4375
`\pi` square inches

Taking
`\pi` =
`(22)/(7)`, then:

Area of tape part = 0.4375 ×
`((22)/(7) )`

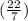
= 1.375 square inches