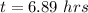Question

Newton's law of cooling is , where is the temperature of an object, is in hours, is a constant ambient temperature, and is a positive constant. Suppose a building loses heat in accordance with Newton's law of cooling. Suppose that the rate constant has the value Assume that the interior temperature is , when the heating system fails. If the external temperature is , how long will it take for the interior temperature to fall to

Answer 1

Complete Question

The complete question is shown on the first uploaded image

Answer:

The time taken is
`t = 6.89 \ hrs`

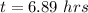
Step-by-step explanation:

From the question we are told that

The value of
`k = 0.15hr^(-1)`

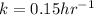
The the interior temperature is
`u(t = 0) = 70 ^oF`

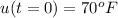
The external temperature is
`T = 11 ^oF`

The required interior temperature is
`u_(t) = 32 ^oF`

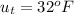
The newton cooling law is

`(du)/(dt) = -k (u -T)`

=>
`(du)/(u-T) = - kdt`

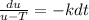
Now integrate both sides we have

`\int\limits (du)/(u-T) =\int\limits - kdt`

`ln (u - T) = -kt + c`

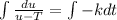
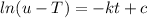
Since c is a constant lnC = c will also give a constant so

`ln (u - T) = -kt + ln C`

=>
`((u -T))/(C) = e^(-kt)`

=>
`u = T + Ce^(-kt)`

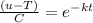
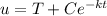
substituting value

`70 = 11 +Ce^(-0* 0.15)`

`C = 59`

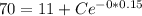
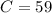
Hence

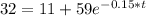
`u_t = 11 + 59 e^(-0.15 *t)`

`32= 11 + 59 e^(-0.15 *t)`

=>
`t = -(ln((21)/(59) ))/(0.15)`

`t = 6.89 \ hrs`