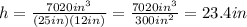Question

Two glass containers are shown. Both are right rectangular prisms. Container 1 is filled with water and Container 2 is empty. Amalia wants to pour all of the water from Container 1 into Container 2. What must the height of Container 2 be in order for it to hold exactly the same amount of water as Container 1?

Answer 1

23.4 inches

Explanation:

The formula for the volume of a rectangular prism is V = l×w×h

First we need to find the volume of Container 1

• The length (l) is 32.5 in
• The width (w) is 18 in
• The height (h) is 12 in
• The volume (V) is ? → what we are solving for
• Plugging in these values into the formula we get: V = (32.5)(18)(12) = 7020 in³

Now that we have the volume for Container 1, we know that Container 2 needs to have the same volume.

Using the same formula as before we can find the height of Container 2

• The length (l) is 25 in
• The width (w) is 12 in
• The height (h) is ? → what we are solving for
• The volume (V) is 7020 in³

We will need to manipulate our formula to solve for h now instead of V

• To do so, we need to divide both sides of the formula by (l×w) to get:
  `h=(V)/(l*w)`
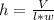
• Plugging in the values that we have to our new formula, we get:
  `h=(7020in^(3) )/((25in)(12in)) =(7020in^3)/(300in^2) = 23.4 in`