Question

Let X denote the number of neutrophils (a special type of white blood cell) in 50randomly sampled white blood cells. If, overall 40% of white blood cells are neutrophils, describethe distribution of X. Compute approximately the probability thatX <15? EXPLAIN THETOOL(S) you are using to compute this probability. Note that you should not be computing theexact probability, as you are not allowed to use a scientific computer that would be necessary tocompute this number.

Answer 1

The probability of X < 15 is 0.054.

Explanation:

The random variable X is defined as the number of neutrophils.

The number of white blood cells sampled is, n = 50.

The proportion of neutrophils is, p = 0.40.

A randomly sampled white blood cells is a neutrophil or not is independent of all the other white blood cells.

The random variable X thus follows a Binomial distribution with parameters n = 50 and p = 0.40.

The probability mass function of the Binomial distribution is:

`P(X=x)={n\choose x}\ p^(x)\ (1-p)^(n-x);\ x=0,1,2,3...`

Compute the probability of X < 15 as follows:

`P (X < 15) =1- P (X \geq 15)`

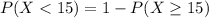
`=1-[\sum\limits^(15)_(i=0){{50\choose x}\ (0.40)^(x)\ (1-0.40)^(50-x)}]\\\\=1-[0+0+...+0.04155]\\\\=1-0.94604\\\\=0.05396\\\\\approx 0.054`

Thus, the probability of X < 15 is 0.054.